Difference between revisions of "Rainwater harvesting: Sizing and modeling"

From LID SWM Planning and Design Guide
Jump to navigation Jump to search
Line 3: Line 3:


===Rapid===
===Rapid===
<p>Five percent of the average annual yield can be calculated using the following equation:<br>
<p>Five percent of the average annual yield can be estimated:<br>
<strong>Y<sub>0.05</sub>= A × C<sub>vol</sub> × R<sub>a</sub> × e × 0.05</strong>
<strong>Y<sub>0.05</sub>= A × C<sub>vol</sub> × R<sub>a</sub> × e × 0.05</strong>
<ul>
<br>Y<sub>0.05</sub> = Five percent of the average annual yield (L)
        <li>Y<sub>0.05</sub> = Five percent of the average annual yield (L)
<br>A = The catchment area ( m<sup>2</sup>)
        <li>A = The catchment area ( m<sup>2</sup>)</li>
<br>C<sub>vol</sub> = The runoff coefficient for the catchment
        <li>C<sub>vol</sub> = The runoff coefficient for the catchment</li>
<br>R<sub>a</sub> = The average annual rainfall depth (mm)
        <li>R<sub>a</sub> = The average annual rainfall depth (mm)</li>
<br>e = The efficiency of the pre-storage filter
        <li>e = The efficiency of the pre-storage filter</li>
    </ul>
</p>
</p>
<p>Five percent of the average annual demand (D<sub>0.05</sub>) is calculated as the product of:<br>
<p>Five percent of the average annual demand (D<sub>0.05</sub>) can be estimated:<br>
<strong>D<sub>0.05</sub> =  P<sub>d</sub> × n × 18.25</strong>  
<strong>D<sub>0.05</sub> =  P<sub>d</sub> × n × 18.25</strong>  
<ul>
<br>D<sub>0.05</sub> = Five percent of the average annual demand (L)
        <li>D<sub>0.05</sub> = Five percent of the average annual demand (L)</li>
<br>P<sub>d</sub> = The daily demand per person (L)
        <li>P<sub>d</sub> = The daily demand per person (L)</li>
<br>n = The number of occupants
        <li>n = The number of occupants</li>
<br>Good catchment selection means that the runoff coefficient (C<sub>vol</sub>) should be 0.9 or greater. Filter efficiency (e) can be reasonably estimated as 0.9 pending manufacturer’s information.</p>
    </ul>
Good catchment selection means that the runoff coefficient (C<sub>vol</sub>) should be 0.9 or greater. Filter efficiency can be reasonably estimated as 0.9 pending manufacturer’s information.</p>
----
----
<p>The following calculation is based upon two criteria:
<p>Then the following calculations are based upon two criteria:
     <ol>
     <ol>
         <li>A design rainfall depth is to be captured entirely by the RWH system.</li>
         <li>A design rainfall depth is to be captured entirely by the RWH system.</li>
Line 28: Line 24:
     </ol>
     </ol>
</p>
</p>
<p><strong>When Y<sub>0.05</sub>/ D<sub>0.05</sub> <0.33</strong>
<p>When Y<sub>0.05</sub>/ D<sub>0.05</sub> < 0.33, the storage volume required (V<sub>S</sub>, in L) can be estimated:<br>
    <br> The total storage volume required (V<sub>S</sub>, in L) is calculated as the product of:
<strong>V<sub>S</sub> = A × C<sub>vol</sub> × R<sub>d</sub> × e</strong><br>
    <ul>
<br>V<sub>S</sub> = Storage volume required (L)
        <li>The catchment area (A, in m<sup>2</sup>)</li>
<br>A = The catchment area (m<sup>2</sup>)
        <li>The design storm runoff coefficient for the catchment (C<sub>vol</sub>)</li>
<br>C<sub>vol</sub> = The design storm runoff coefficient for the catchment
        <li>The design storm rainfall depth (R<sub>d</sub>, in mm), and </li>
<br>R<sub>d</sub> = The design storm rainfall depth (mm), and  
        <li> the efficiency of the pre-storage filter (e). </li>
<br>e = The efficiency of the pre-storage filter.  
    </ul>
 
</p>
</p>
<p><strong>When 0.33 < Y<sub>0.05</sub>/ D<sub>0.05</sub> <0.7</strong>
<p>When 0.33 < Y<sub>0.05</sub>/ D<sub>0.05</sub> < 0.7, the total storage required can be estimated by adding Y<sub>0.05</sub>: <br>
    <br> The total storage required is the sum of V<sub>S</sub> and Y<sub>0.05</sub>. </p>
<strong>Total storage = V<sub>S</sub> + Y<sub>0.05</sub></strong>
 
</p>
----
</div>
</div>
<div class="col-md-4">
<div class="col-md-4">

Revision as of 19:22, 11 July 2017

Rapid[edit]

Five percent of the average annual yield can be estimated:
Y0.05= A × Cvol × Ra × e × 0.05
Y0.05 = Five percent of the average annual yield (L)
A = The catchment area ( m2)
Cvol = The runoff coefficient for the catchment
Ra = The average annual rainfall depth (mm)
e = The efficiency of the pre-storage filter

Five percent of the average annual demand (D0.05) can be estimated:
D0.05 = Pd × n × 18.25
D0.05 = Five percent of the average annual demand (L)
Pd = The daily demand per person (L)
n = The number of occupants
Good catchment selection means that the runoff coefficient (Cvol) should be 0.9 or greater. Filter efficiency (e) can be reasonably estimated as 0.9 pending manufacturer’s information.

Then the following calculations are based upon two criteria:

  1. A design rainfall depth is to be captured entirely by the RWH system.
  2. The average annual demand (D) is greater than the average annual yield (Y) from the catchment.

When Y0.05/ D0.05 < 0.33, the storage volume required (VS, in L) can be estimated:
VS = A × Cvol × Rd × e

VS = Storage volume required (L)
A = The catchment area (m2)
Cvol = The design storm runoff coefficient for the catchment
Rd = The design storm rainfall depth (mm), and
e = The efficiency of the pre-storage filter.

When 0.33 < Y0.05/ D0.05 < 0.7, the total storage required can be estimated by adding Y0.05:
Total storage = VS + Y0.05

<panelInfo>

  • Schematic diagram of the inputs and outputs to a rainwater harvesting cistern

</panelInfo>

STEP Rainwater Harvesting Tool[edit]

The Sustainable Technologies Evaluation Program have produced a rainwater harvesting design and costing tool specific to Ontario. The tool is in a simple to use Excel format and is free to download.

In a study of three sites in Ontario, STEP found the annual Cvol of the rooftops to be around 0.8 [1]. This figure includes losses to evaporation, snow being blown off the roof, and number of overflow events.

<panelWarning> STEP Rainwater Harvesting Tool </panelWarning>

The Treatment Train Tool[edit]

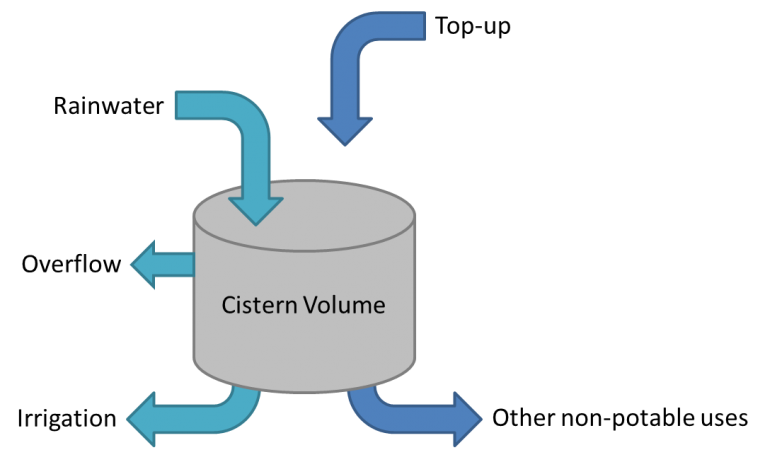
Once the size of cistern has been determined, it can easily be modelled in many open source and proprietary applications.

In addition to the cistern size, this watershed scale modelling requires input information about draw down. time i.e. the rate of use.

<btnPrimary>The Treatment Train Tool</btnPrimary>

  1. REDIRECT Special:ArticleFeedbackv5

The land draining to a single reference point (usually a structural BMP); similar to a subwatershed, but on a smaller scale.

The portion of water precipitated onto a catchment area, which then flows as surface discharge from the catchment area past a specified point.

Water from rain, snow melt, or irrigation that flows over the land surface.

Rainwater harvesting.

The practice of intercepting, conveying and storing rainwater for future use. Captured rainwater is typically used for outdoor non-potable water uses such as irrigation, or in the building to flush toilets.

Tank used to store rainwater (typically roof runoff) for later use.

Sustainable Technologies Evaluation Program

Abiotic transfer of water vapour to the atmosphere.

Retrieved from "https://wikidev.sustainabletechnologies.ca/index.php?title=Rainwater_harvesting:_Sizing_and_modeling&oldid=1665"