Difference between revisions of "Grading"
Jenny Hill (talk | contribs) m |
Jenny Hill (talk | contribs) |
||
| (23 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
[[File:Theta.jpg| | {{TOClimit|2}} | ||
==Slope gradient== | ==Expressing slope== | ||
Slope gradients are | {{float right|[[File:Theta.jpg|border|500 px]]}} | ||
===Slope gradient=== | |||
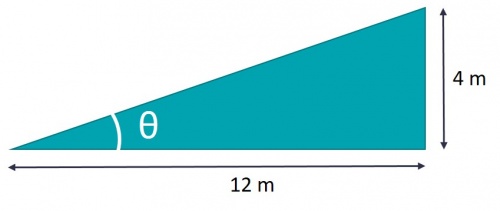
Slope gradients are are common form of expression for steeply sloped areas like the sides of [[swales]]. Expressed as Y:X where Y is a single unit of rise and X is the corresponding run. It can be calculated by dividing the rise by the run: | |||
e.g.:<math>\frac{rise}{run}=\frac{4\ m}{12\ m}=\frac{1}{3};we\ say\ the\ slope\ =1:3\ or\ 1\ in\ 3</math> | e.g.:<math>\frac{rise}{run}=\frac{4\ m}{12\ m}=\frac{1}{3};we\ say\ the\ slope\ =1:3\ or\ 1\ in\ 3</math> | ||
==Slope percentage== | ===Slope percentage=== | ||
Slope percentage is calculated similarly by dividing rise by run, but then multiplying by 100 to get a percentage: | Slope percentage is calculated similarly by dividing rise by run, but then multiplying by 100 to get a percentage: | ||
e.g.:<math>slope=\left (\frac{rise}{run}\right )\times 100=\left(\frac{4\ m}{12\ m}\right)\times 100= 33\%</math> | e.g.:<math>slope=\left (\frac{rise}{run}\right )\times 100=\left(\frac{4\ m}{12\ m}\right)\times 100= 33\%</math> | ||
'''But''' for many calculations the ''rise/run'' isn't multiplied by 100, and instead is left as a decimal. e.g. 0.33 in the example above. | |||
==Slope in degrees== | ===Slope in degrees=== | ||
Expressing a slope in degrees requires using the '' | Expressing a slope in degrees requires using the ''tangent'' trigonometric function. | ||
e.g.:<math>\tan \theta =\left ( \frac{4\ m}{12\ m} \right ); \theta =\tan^{-1}\left ( 0.33 \right )=18.3^{\circ}</math> | e.g.:<math>\tan \theta =\left ( \frac{4\ m}{12\ m} \right ); \theta =\tan^{-1}\left ( 0.33 \right )=18.3^{\circ}</math> | ||
<ref>Ontario. O. Reg. 191/11: INTEGRATED ACCESSIBILITY STANDARDS, 2011. https://www.ontario.ca/laws/regulation/r11191.</ref> | '''[[Slope_table| Quick reference table]]''' | ||
==Normal range of slopes== | |||
The Accessibility for Ontarians with Disabilities Act<ref>Ontario. O. Reg. 191/11: INTEGRATED ACCESSIBILITY STANDARDS, 2011. https://www.ontario.ca/laws/regulation/r11191.</ref> provides a bare minimum to which public spaces must be designed. e.g. cross slope not to exceed 2 %. | |||
Consider designing landscapes to universal design principals instead<ref>Universal Design.com. 2018. “The Leading Source for News and Information on Universal Design - The Leading Source for News and Information on Universal Design.” 2018. http://www.universaldesign.com/.</ref><ref>Institute for Human Centered Design. 2018. “Principles.” 2018. https://www.humancentereddesign.org/inclusive-design/principles.</ref>. | |||
{|class= "wikitable" "style=text-align: center;" | {|class= "wikitable" "style=text-align: center;" | ||
| Line 51: | Line 59: | ||
|Planted banks||up to 100||up to 50 | |Planted banks||up to 100||up to 50 | ||
|} | |} | ||
==Freeboard== | |||
{{:Freeboard}} | |||
==Additional Resources== | |||
*[[File:Huang 2012.pdf]] | |||
*[[File:Matusik 2004.pdf]] | |||
*[[File:Tiner 2014.pdf]] | |||
*http://www.slopemeter.com/images/Slope_Conversion_Tables.xls - external resource | |||
---- | |||
[[Category: Calculations]] | |||
Latest revision as of 17:55, 22 December 2018
Expressing slope
Slope gradient
Slope gradients are are common form of expression for steeply sloped areas like the sides of swales. Expressed as Y:X where Y is a single unit of rise and X is the corresponding run. It can be calculated by dividing the rise by the run: e.g.:
Slope percentage
Slope percentage is calculated similarly by dividing rise by run, but then multiplying by 100 to get a percentage: e.g.: But for many calculations the rise/run isn't multiplied by 100, and instead is left as a decimal. e.g. 0.33 in the example above.
Slope in degrees
Expressing a slope in degrees requires using the tangent trigonometric function. e.g.:
Normal range of slopes
The Accessibility for Ontarians with Disabilities Act[1] provides a bare minimum to which public spaces must be designed. e.g. cross slope not to exceed 2 %.
Consider designing landscapes to universal design principals instead[2][3].
| Use | Extreme range (%) | Desirable range (%) |
|---|---|---|
| Public Streets | 0.5 - 10 | 1 - 8 |
| Private streets | 0.5 - 20 | 1 - 12 |
| Service lanes | 0.5 - 15 | 1 - 10 |
| Parking areas | 0.5 - 8 | 1 - 5 |
| Parking ramps | up to 20 | up to 15 |
| Collector walks | 0.5 - 12 | 1 - 8 |
| Entrance walks | 0.5 - 8 | 1 - 4 |
| Pedestrian ramps | up to 12 | up to 8 |
| Stairs | 25 - 50 | 33 - 50 |
| Game courts | 0.5 - 2 | 0.5 - 1.5 |
| Paved gutters | 0.25 - 100 | 1 - 50 |
| Grassed swales | 0.5 - 15 | 2 - 10 |
| Terraces and sitting areas | 0.5 - 3 | 1 - 2 |
| Grassed banks | up to 50 | up to 33 |
| Planted banks | up to 100 | up to 50 |
Freeboard
- In swales conveying flowing water a freeboard of 300 mm is generally accepted as a good starting point.
- In bioretention the freeboard is the difference between the invert elevation of the overflow structure and the inlet. 150 mm will suffice, so long as the inlet will not become inundated during design storm conditions.
- In above grade stormwater planters, the equivalent dimension would be the difference between the invert elevation of the overflow structure and the lip of the planter (150 mm minimum)
Additional Resources
- File:Huang 2012.pdf
- File:Matusik 2004.pdf
- File:Tiner 2014.pdf
- http://www.slopemeter.com/images/Slope_Conversion_Tables.xls - external resource
- ↑ Ontario. O. Reg. 191/11: INTEGRATED ACCESSIBILITY STANDARDS, 2011. https://www.ontario.ca/laws/regulation/r11191.
- ↑ Universal Design.com. 2018. “The Leading Source for News and Information on Universal Design - The Leading Source for News and Information on Universal Design.” 2018. http://www.universaldesign.com/.
- ↑ Institute for Human Centered Design. 2018. “Principles.” 2018. https://www.humancentereddesign.org/inclusive-design/principles.
- ↑ Strom, S., Nathan, K., Woland, J. Site Engineering for Landscape Architects (6th Ed.) 2013, Wiley and Sons